Sidebar. The Maxwell-Boltzmann distribution of molecular velocities
Think of molecules in, say, a cubic meter of air around your head, colliding with each other, changing both direction and speed at great frequency – about 6 billion times a second. With all these changes, there are great regularities. Barring some real disturbance, the parcel of air has a well-defined temperature. It has a well-defined pressure, with a highly invariant number of molecular hits imparting momentum to your head. It has a well-defined diffusivity of its own molecules and of any odorants you might add. The distribution of speeds and of collisions fits the rate of chemical reactions between molecules extremely well. This is all a result of the molecules achieving an exquisitely regular distribution of velocities (speeds and directions) – the Maxwell-Boltzmann distribution.
Figuring out the speed distribution. It would be impossible to calculate the ever-changing velocities of individual molecules to “prove” this distribution, although the kinetic theory of gases has impressive theorems about how groups of molecules move into other groups with no net change in population of groups. (Note that molecular dynamics simulations on high-end computers are used to model reactions and structures of up to thousands of atoms and molecules, but there’s no point in doing it for a simple gas of 1023 molecules.) The basic postulate is that molecules adopt a distribution of velocities that represents the maximal disorder at a given temperature (total energy, therefore), density, and molecular masses. The derivation involves a significant number of concepts in physics and associated algebraic calculations; these can be followed in many online postings.
There are two ways, at least, to describe the distribution of velocities. One is as velocities in the three Cartesian directions, x, y, z. Since these are all equivalent, in the absence of some directional force such as a magnetic field on magnetic molecules, it’s simpler to use the speeds alone. (If you haven’t had a mechanics course, velocity is a vector, a speed and a direction.) The result of the famous derivations is expressed as a probability, f(v) (read as “ f of v”) of finding molecules with speeds between any chose value, v, and a nearby increment, dv – say, 300 meters per second and 300.5. In calculus we use the limit of a vanishing interval, but this is not critical to comprehending the expression:
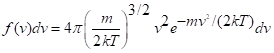
This equation has a number of features. One is that the distribution falls off quickly, as the negative exponential, for high speeds. We note that mv2/2 is the kinetic energy of a molecule and kT is also an energy; the average kinetic energy of the molecules can be shown by integration to be (3/2)kT. Molecules with high kinetic energy are rare. The factor v2 comes in because there are more and more ways of moving at high energy.
We may plot this for some notional mass of the individual molecules, m, and temperature, T. The Boltzmann constant, k = 1.38×10-23 m2 kg s-2 K-1 , is universal, independent of the nature of the molecules and all else. Let’s take the dominant molecules is air, nitrogen, with masses of 28 atomic units – that’s 28 grams or 0.028 kg per mole. Dividing by Avogadro’s number, N0 = 6.024×1023, we get the mass of the individual N2 molecule as 4.65×10-26 kg, to three significant figures. Take T as room temperature, 20°C = 293K. Plugging these values into Excel, for example, we get this plot:
The most probable speed, at the peak, can seem surprising, quite peppy, at just over 400 ms-1 (880 mph). Of course, that’s consistent with the speed of sound being about 330 ms-1 (there’s some thermodynamics in that calculation). The peak speed, in fact, is 
. The mean (average) speed is a bit different, by a factor 2/ 
or 1.13 times larger. The speed at the mean kinetic energy is larger by a factor 
= 1.22.
What about other molecules in the same parcel of air, say, oxygen (O2) and argon (Ar)? They’re heavier. The shape of the curves for them is exactly the same, just shifted to lower speeds. For oxygen that’s heavier by a factor of 32/28 or 14% larger the peak speed is 7% smaller. For argon with its mass of 40 amu it’s 14% smaller. The presence of other, faster, lighter N2 molecules makes no difference in the distributions for these molecules. (Note that argon is pretty abundant in the air, at 0.93% of all the molecules in dry air. It accumulated as the product of decay of radioactive potassium-40… remember the “reference” value of a potassium-rich banana as about 1/10,000,000th of a Sievert for exposure to radioactivity.)
Moving fast enough to escape a planet’s gravity. This is a concept critical to habitability. Mars has a gravitational force at its surface only 0.38 as large as the Earth has. It was unable to hold onto the hydrogen atoms split off from water by solar ultraviolet radiation, so it became a dry planet. This topic is developed in the main text.
Molecules are not always stable or inert to change. Flammable hydrocarbons such as gasoline vapors undergo rare reactions with oxygen molecules at room temperature. At increasing temperatures, the reaction takes on a chain-reaction form and spontaneous ignition occurs. Some compounds are even more susceptible. Carbon disulfide ignites in air at 90°C, less than the boiling point of water. I had some of this (and many other dangerous chemicals) as a kid. I still have my eyes, hands, and liver, luckily. People who work in viscose rayon plants are less lucky. CS2 is the main solvent.
There are other modes of motion besides translation (kinetic energy) in many molecules. Oxygen and nitrogen molecules, and CO2, rotate freely at room temperature. They store a major part of their thermal energy in rotation. The molecules can also vibrate, but quantum-mechanical phenomena keep vibrational energy low until high temperatures are reached. At room temperature vibrations in O2 store only about 1/2000th as much energy as translation.
At very low temperatures, quantum mechanics has a lot to say. The derivation of the Maxwell-Boltzmann distribution in classical mechanics viewed the molecules as distinguishable. We could notionally mark each one with its own little license plate. Quantum mechanics says they are indistinguishable. That makes a big difference at low temperatures, at which condition molecules go toward accumulating in the same energy state. Different molecules can act as either fermions, unable to be in the same state at the same time, or boson, favoring occupying the same state. Oxygen (O2) is a boson; helium-3 is a fermion.
The idea that the pressure exerted on a body is constant in a fluid at a fixed temperature and pressure has its limitations. A small body sees a small set of molecules colliding with it. These vary in speed. At times, the set of molecules hitting a small body is more energetic than average, and less energetic at other times. As a consequence, small bits of matter such as tiny pollen grains show Brownian motion. It’s a fast random walk in space that initially made people think that it was a “vital”motion, but inert dust motes move the same way. Einstein showed the theory of Brownian motion rests on the distribution of molecular velocities. It accounted for 1/3 of his Nobel Prize nomination.